Pentagons: A Five-Sided Polygon
A pentagon is a polygon with five straight sides and five angles. It is a fascinating geometric shape that has intrigued mathematicians and architects for centuries. Pentagons have unique properties and characteristics that set them apart from other polygons, making them a subject of study in various fields.
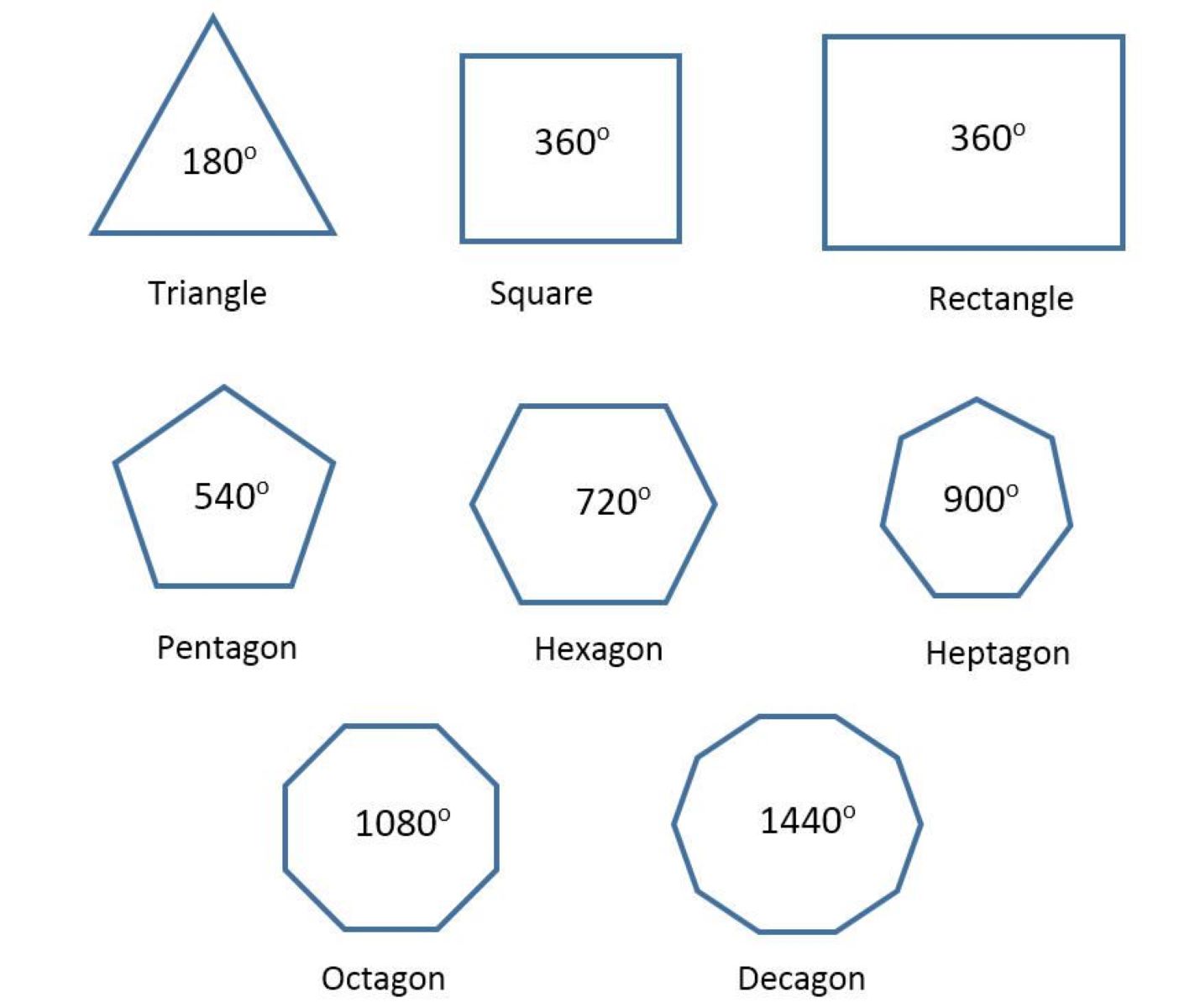
One of the most distinctive features of pentagons is their symmetry. A regular pentagon has equal side lengths and equal interior angles, making it a geometrically balanced shape. Its interior angles measure 108 degrees each, totaling 540 degrees when combined. This symmetry is not only visually pleasing but also mathematically significant.
Another interesting property of pentagons is their versatility in tiling. Pentagonal tiles can fill a plane without any gaps or overlaps, known as pentagonal tiling. This unique attribute has been utilized in architecture and design, creating intricate patterns and aesthetically pleasing structures.
Pentagons come in different variations, each with its own set of characteristics. For instance, a regular pentagon has all sides and angles equal, while an irregular pentagon has different side lengths and angles. There are also various types of irregular pentagons, such as concave and convex pentagons, distinguished by their internal angle measurements.
The application of pentagons extends beyond the realm of geometry. In nature, some organisms exhibit pentagonal symmetry, like certain sea urchins and starfish. Additionally, pentagonal shapes can be found in everyday objects, such as traffic signs, soccer balls, and house floor plans.
Understanding the Properties of Pentagons
In order to fully comprehend the intricacies of pentagons, it is essential to delve into their properties and understand how they differ from other polygons. By exploring the characteristics of pentagons, we gain insight into their unique geometric structure.
One prominent feature of pentagons is the sum of their interior angles. In any polygon, the sum of the interior angles is determined by the formula (n-2) * 180 degrees, where n represents the number of sides. For pentagons, with five sides, the sum of the interior angles is (5-2) * 180 degrees, equaling 540 degrees. This property holds true for all pentagons, whether regular or irregular.
The exterior angles of a pentagon also follow a specific pattern. Each exterior angle is formed by extending one side of the pentagon outward, and it is equal to the supplementary angle of the corresponding interior angle. In a regular pentagon, where all interior angles are congruent, each exterior angle measures 72 degrees. In irregular pentagons, the exterior angles can vary in value depending on the lengths of the sides and the arrangement of the angles.
Pentagons also possess rotational symmetry. A regular pentagon can be rotated around its center point by multiples of 72 degrees, resulting in the exact same shape. This rotational symmetry highlights the balance and harmony inherent in pentagonal geometry.
Another fascinating property of pentagons is their relationship with the Golden Ratio. The Golden Ratio, often represented by the Greek letter phi (Φ), is a mathematical constant approximately equal to 1.6180339887. The Golden Ratio frequently appears in pentagons, as the ratio of the length of the longer side to the shorter side is close to this constant. This unique connection adds a layer of beauty and intrigue to the study of pentagons.
By understanding the properties of pentagons, we gain a deeper appreciation for their elegance and significance in mathematics and geometry. These properties provide a foundation for exploring the various types of pentagons and their applications in real-life scenarios.
Types of Pentagons and Their Characteristics
Pentagons come in various types, each with its own distinct characteristics. These variations in shape, size, and angle measurements contribute to the diversity and versatility of pentagonal geometry. Let’s explore some of the common types of pentagons along with their unique properties.
1. Regular Pentagons: A regular pentagon is a polygon with all sides and angles equal. It has five line segments of the same length and all internal angles measuring 108 degrees. Regular pentagons possess rotational symmetry, meaning they can be rotated by multiples of 72 degrees around their center point and still maintain their original shape.
2. Irregular Pentagons: In contrast to regular pentagons, irregular pentagons have different side lengths and angle measurements. The angles in an irregular pentagon can vary, creating an asymmetrical shape. Irregular pentagons come in a wide range of forms and can be concave or convex depending on the arrangement of their internal angles.
3. Convex Pentagons: A convex pentagon has no interior angles greater than 180 degrees. It is a polygon without any inwardly projecting sides. Convex pentagons are often seen in architectural designs, where their stable and structurally sound shape is essential.
4. Concave Pentagons: Unlike convex pentagons, concave pentagons have at least one interior angle greater than 180 degrees. They possess one or more indentations or “caves” in their shape. Concave pentagons, with their unique contours, add artistic flair to various designs and patterns.
5. Golden Ratio Pentagons: The Golden Ratio, also known as the Divine Proportion, is a mathematical concept that occurs in nature and art. Golden Ratio pentagons have a specific ratio between the longer and shorter sides, which closely approximates the Golden Ratio (approximately 1.618). This unique connection to the Golden Ratio adds an element of aesthetic intrigue to these pentagons.
Understanding the characteristics of different types of pentagons allows us to appreciate their diverse applications in both theoretical mathematics and practical fields. From architectural structures to artistic designs, pentagons offer endless possibilities for creativity and exploration.
Exploring the Application of Pentagons in Real Life
Pentagons, with their unique properties and versatile shapes, find applications in various aspects of real life. They can be observed in architecture, design, nature, and everyday objects, showcasing the practicality and aesthetic appeal of this five-sided polygon.
One of the most well-known examples of pentagons in real life is in the design of soccer balls. The iconic black and white pattern on soccer balls consists of 12 regular pentagons and 20 regular hexagons arranged in a spherical shape. This configuration ensures that the ball maintains a balanced and predictable trajectory when kicked.
Pentagons are also prevalent in architectural designs. Their symmetry and stability make them ideal for creating visually appealing structures. For instance, the United States Pentagon building in Arlington, Virginia, is a famous example of pentagonal architecture. Its distinct five-sided shape not only represents strength and order but also maximizes space utilization and efficiency.
Pentagonal tiling, a pattern formed using regular pentagons, is another practical application of pentagons. This tiling technique is often used in interior design and flooring, creating captivating and patterned surfaces. It can be seen in various places such as shopping malls, airports, and hotel lobbies.
In nature, some organisms exhibit pentagonal symmetry. Starfish and certain types of sea urchins have five-fold radial symmetry, with their body parts arranged in a pentagonal pattern. This natural occurrence highlights the significance and prevalence of pentagons in the biological world.
Moreover, pentagons have found application in the field of optics. In the design of camera lenses, five-sided apertures are sometimes used to control the amount and shape of light entering the lens. The pentagonal shape helps to create aesthetically pleasing bokeh effects, adding a sense of depth and artistic appeal to photographs.
From sports equipment to architecture, interior design to nature, and optics to everyday objects, the application of pentagons in real life is remarkably diverse. This showcases the wide-ranging impact and significance of this unique polygon.
Hexagons: A Six-Sided Polygon
A hexagon is a polygon with six straight sides and six angles. It is a widely recognized geometric shape due to its prevalence in nature, art, and everyday objects. Hexagons possess unique properties and characteristics that make them fascinating objects of study in geometry.
One notable feature of hexagons is their exceptional efficiency in packing. When placing hexagons side by side, they tessellate perfectly without leaving any gaps or overlaps. This exceptional property is seen in the honeycombs made by bees, where hexagonal cells are formed to maximize storage space while using the least amount of material.
Another intriguing aspect of hexagons is their balance of symmetry and versatility. Regular hexagons have all sides and angles equal, making them geometrically balanced. Each interior angle of a regular hexagon measures 120 degrees, resulting in a sum of interior angles equal to 720 degrees. This symmetry lends itself to the creation of aesthetically pleasing patterns and designs.
Hexagons have a close connection to the concept of the Golden Ratio. The ratio of the length of one side of a regular hexagon to the length of its radius is equal to the golden ratio, approximately 1.618. This geometric relationship adds an element of harmony and beauty to hexagonal structures in art and design.
The practicality of hexagons is evident in their numerous real-life applications. In the field of construction, hexagonal tiles are commonly used for flooring and wall designs. The interlocking pattern of hexagons creates a visually captivating surface while ensuring structural integrity.
Hexagonal shapes are also prevalent in the natural world. For example, many crystals exhibit a hexagonal lattice structure, such as quartz and snowflakes. This natural occurrence demonstrates the inherent efficiency and stability of hexagons in forming intricate and symmetrical arrangements.
Furthermore, hexagons are often used in engineering and manufacturing processes. The hexagon’s regularity and strength make it an excellent choice for creating nuts, bolts, and screws. The hexagonal shape allows for better grip and torque, making it easier to tighten and secure objects.
Hexagons are not only geometrically fascinating but also widely applicable in various fields. From nature’s intricate patterns to human-made structures and industrial applications, hexagons continue to captivate our imaginations and prove their versatility.
Key Properties and Characteristics of Hexagons
Hexagons, with their six sides and six angles, possess a unique set of properties and characteristics that distinguish them from other polygons. These properties contribute to their structural stability, aesthetic appeal, and practical applications in various fields. Let’s explore some of the key properties and characteristics of hexagons.
One of the most prominent features of hexagons is their interior angle measurement. In a regular hexagon, all interior angles are equal and measure 120 degrees. This uniformity creates a visually pleasing symmetry and balance within the shape.
Hexagons also exhibit rotational symmetry. A regular hexagon can be rotated around its center point by multiples of 60 degrees while still maintaining its original shape. This rotational symmetry enhances the aesthetic appeal of hexagons and allows for creative designs and patterns.
Another intriguing property of hexagons is their ability to tessellate, or tile, without any gaps or overlaps. When hexagons are arranged next to one another, they create a seamless pattern. This efficient packing property is observed in nature, such as in beehives, and in various human-made structures, including tiling designs.
The relationship between the side length and the radius of a regular hexagon is significant. The ratio of the side length to the radius is equal to the square root of 3 (approximately 1.732). This geometric relationship adds a touch of elegance and harmony to hexagonal shapes, making them visually appealing in art and design.
Hexagons possess exceptional strength and stability due to the distribution of forces along their sides. The evenly distributed angles and sides of a hexagon allow for maximum load-bearing capacity, making them ideal for structural applications in architecture and engineering.
The close connection between hexagons and the concept of the Golden Ratio is also noteworthy. The ratio of the length of one side of a regular hexagon to its radius is approximately equal to the Golden Ratio (approximately 1.618). This mathematical relationship further enhances the aesthetic appeal of hexagons and the sense of proportion in their design.
From their symmetrical angles to their efficient tiling properties, hexagons exhibit a range of characteristics that make them incredibly versatile and fascinating. Whether found in nature, art, or industry, hexagons continue to inspire and prove their significance in various fields.
Different Types of Hexagons and Their Uses
Hexagons, with their distinctive six sides and angles, come in various types, each with its own unique characteristics and practical applications. Let’s explore some of the different types of hexagons and how they are utilized in different fields.
1. Regular Hexagons: Regular hexagons have all sides and angles equal. They possess rotational symmetry, allowing them to be rotated by multiples of 60 degrees while maintaining their shape. Regular hexagons are commonly seen in architecture, where their balanced and symmetrical form creates visually pleasing designs in building facades and floor patterns.
2. Irregular Hexagons: Irregular hexagons have sides of varying lengths and angles that are not all equal. These hexagons offer more design flexibility and are often used in artistic and creative applications. Irregular hexagons can be found in modern art installations, decorative tiles, and logo designs.
3. Convex Hexagons: Convex hexagons have no internal angles greater than 180 degrees. They are typically seen in engineering and manufacturing applications. The regularity of convex hexagons makes them suitable for creating nuts, bolts, and other fastening mechanisms, as their shape allows for easy grip and secure fastening.
4. Concave Hexagons: Concave hexagons have at least one internal angle greater than 180 degrees. The unique shape of concave hexagons adds a sense of intrigue and artistic appeal. They are often used in architectural design to create unconventional building facades, sculptures, and unique structural elements.
5. Honeycomb Hexagons: In nature, hexagons are prevalent in the form of honeycombs created by bees. The hexagonal cells of a honeycomb maximize storage space, allowing bees to store honey and the development of their larvae efficiently. This natural hexagonal structure provides inspiration for efficient storage solutions and architectural designs.
Hexagons also find applications in the field of optics. Hexagonal lens arrays are used in various optical devices, such as cameras, to reduce chromatic aberration and improve image quality. The hexagonal shape allows for better coverage and distribution of light, resulting in sharper and clearer images.
Furthermore, hexagons are widely used in tessellations and tiling patterns. The interlocking nature of hexagonal tiles ensures a seamless and visually appealing surface. Hexagonal tiles are commonly found in kitchens, bathrooms, and other interior spaces for their aesthetic appeal and ease of installation.
From regular and irregular hexagons to convex and concave forms, the different types of hexagons offer diverse applications across various fields. Their structural integrity, tessellation properties, and aesthetic appeal make them valuable in architecture, design, manufacturing, and more.
Real-Life Examples of Hexagons
Hexagons, with their unique properties and versatile nature, can be observed in numerous real-life examples across different fields and settings. From nature to construction, here are some notable real-life instances where hexagons are present:
1. Beehives: One of the most renowned examples of hexagons in nature is the honeycomb structure created by bees. Bees construct hexagonal cells to store honey and raise their young. This efficient use of space allows bees to maximize storage capacity while using the least amount of wax material.
2. Tiling Patterns: Hexagonal tiling patterns are commonly used in interior design and architecture. Hexagonal tiles fit together seamlessly, creating visually captivating floors, walls, and backsplashes. The beehive-inspired pattern adds a unique touch to spaces, combining both aesthetics and functionality.
3. Snowflakes: Snowflakes, which form through the crystallization of water vapor in freezing temperatures, often exhibit intricate hexagonal patterns. Each snowflake has a unique hexagonal structure, highlighting the natural beauty and symmetry found in these frozen formations.
4. Soccer Balls: The classic pattern on a soccer ball consists of black and white hexagons and pentagons. The regular hexagons create the majority of the ball’s surface, forming a tight and visually appealing mesh. This pattern ensures that the ball’s surface is even and facilitates predictable ball movement during play.
5. Architectural Design: Hexagons can be found in architectural designs, offering structural stability, balance, and aesthetics. Incorporating hexagons into building facades, windows, or interior elements adds interest and visual appeal to architectural structures. The regularity and symmetry of hexagons contribute to pleasing and harmonious designs.
6. Industrial Applications: Hexagonal shapes are widely utilized in manufacturing and engineering. For example, nuts and bolts often have hexagonal heads, making them easy to grip and tighten securely. The six-sided structure allows for efficient application of torque, facilitating the assembly and disassembly of various components.
7. Optics: Hexagonal lens arrays are used in optical devices such as cameras and telescopes. Hexagons provide better coverage and distribution of light, reducing aberrations and improving image quality. This design ensures precise and clear imaging in various optical systems.
8. Geological Structures: Basalt columns, such as the famous Giant’s Causeway in Northern Ireland, exhibit hexagonal shapes. These natural geological formations, resulting from the cooling and cracking of volcanic lava, showcase the inherent hexagonal properties found in the earth’s crust.
From natural formations like snowflakes and beehives to human-made structures like soccer balls and architectural design elements, hexagons are prevalent in various real-life examples. Their efficiency, strength, and aesthetic appeal make them a versatile and fascinating geometric shape.
Dodecagons: A Twelve-Sided Polygon
A dodecagon is a polygon with twelve straight sides and twelve angles. Its name is derived from the Greek words “dodeka” meaning twelve, and “gon” meaning angle. Dodecagons are intriguing geometric shapes that have unique properties and applications in various fields.
One notable characteristic of dodecagons is their symmetry. A regular dodecagon has twelve equal sides and twelve equal interior angles, each measuring 150 degrees. This symmetry provides an aesthetically pleasing and balanced shape, making it visually appealing in architectural designs and decorative patterns.
Dodecagons possess rotational symmetry, meaning they can be rotated around their center by multiples of 30 degrees while preserving their original shape. This rotational property allows for creative and versatile use in designs and tessellations.
The sum of the interior angles of a dodecagon is 1800 degrees. The formula used to calculate the sum of the interior angles of any polygon is (n-2) * 180 degrees, where n represents the number of sides. With twelve sides, the sum of the interior angles of a dodecagon is (12-2) * 180 degrees, equaling 1800 degrees.
Dodecagons also exhibit radial symmetry. Each side of a regular dodecagon can be traced to a central point, creating a star-like pattern. This symmetry is often seen in art and decorative motifs, adding an element of intrigue and complexity.
In real-life applications, dodecagons can be found in architectural elements such as windows, floor tiles, and ornamentations. The regularity and balance of dodecagons make them visually appealing, adding elegance to building facades and interior spaces.
Dodecagons are also utilized in the design of clocks and timepieces. The twelve-hour division of clocks is often based on the dodecagonal shape. The symmetrical design of a dodecagon allows for an even distribution of hours and ensures accuracy in timekeeping.
The structure of soccer balls, specifically the panels that form the ball, also incorporates dodecagons. This design ensures equal distribution of stitching points and maintains the ball’s shape during play.
Overall, dodecagons with their twelve sides and distinct properties offer a fascinating glimpse into the world of polygons. Their symmetrical and rotational features make them ideal for architectural design, timekeeping devices, and sporting equipment.
Unveiling the Unique Characteristics of Dodecagons
Dodecagons, with their twelve sides and twelve angles, possess a set of distinct characteristics that make them intriguing polygons. These unique properties contribute to their aesthetic appeal, mathematical significance, and applications in various fields.
One of the notable characteristics of dodecagons is their symmetry. A regular dodecagon has twelve equal sides and twelve equal interior angles, each measuring 150 degrees. This symmetrical feature creates a visually pleasing and well-balanced shape, making it suitable for architectural designs, decorative elements, and artistic patterns.
Rotational symmetry is another fascinating aspect of dodecagons. They can be rotated around their center by multiples of 30 degrees while maintaining their original shape. This rotational property enables the creation of intricate designs, tessellations, and symmetrical arrangements, adding versatility to their applications in art and mathematics.
The sum of the interior angles of a dodecagon is 1800 degrees. This can be calculated using the formula (n-2) * 180 degrees, where n represents the number of sides. For a dodecagon with twelve sides, the sum of its interior angles is (12-2) * 180 degrees, equaling 1800 degrees. The consistent sum of interior angles is a defining characteristic of dodecagons.
Another noteworthy feature of dodecagons is their radial symmetry. Each side of a regular dodecagon can be traced to a central point, creating a star-like pattern. This radial symmetry lends itself to captivating designs and decorative motifs, making dodecagons visually appealing in various contexts.
Real-life applications of dodecagons can be found in architectural elements such as windows, floor tiles, and ornamental details. The regularity and balance of dodecagons make them visually appealing, enhancing the aesthetics of building facades and interior spaces.
Dodecagons also play a role in timekeeping devices. The twelve-hour divisions on clocks and watches are often based on the dodecagonal shape. The symmetrical dodecagon ensures an even distribution of hours and maintains accuracy in time measurement.
In the realm of sports, dodecagons are incorporated into the design of soccer balls. The panels that form the structure of the ball often utilize dodecagons, ensuring equal distribution of stitching points and maintaining the ball’s round shape during play.
With their distinct symmetry, rotational properties, consistent sum of interior angles, and radial symmetry, dodecagons showcase an array of unique characteristics. These attributes contribute to their applications in architectural design, timekeeping devices, sports equipment, and other areas where their aesthetic appeal and mathematical significance are valued.
Various Types of Dodecagons and Their Functions
Dodecagons, with their twelve sides and twelve angles, come in various types, each with its own distinct characteristics and applications. Explore the different types of dodecagons and their unique functions in various fields.
1. Regular Dodecagons: Regular dodecagons have twelve equal sides and twelve equal angles, each measuring 150 degrees. Their symmetry and balanced proportions make them aesthetically pleasing. In architecture, regular dodecagons are utilized in decorative elements, windows, and floor tiles, adding an elegant touch to building designs and interior spaces.
2. Star-Shaped Dodecagons: Star-shaped dodecagons, also known as stellated dodecagons, are formed by extending each side of a regular dodecagon to create inward-pointing triangles. This results in a striking star-like appearance. Star-shaped dodecagons often serve as decorative motifs in artwork, logo designs, and intricate patterns.
3. Irregular Dodecagons: Irregular dodecagons have sides of varying lengths and angles that are not all equal. These dodecagons offer more design flexibility and are often used in artistic and creative applications. Irregular dodecagons can be found in abstract art, architectural ornamentations, and decorative elements, allowing for unique and visually intriguing designs.
4. Skew Dodecagons: Skew dodecagons are dodecagons whose sides are not parallel or perpendicular to each other. Their unique angles and skewed appearance create dynamic and visually captivating shapes. Skew dodecagons find applications in geometric sculptures, modern architectural facades, and contemporary art installations.
5. Concave Dodecagons: Concave dodecagons have at least one interior angle greater than 180 degrees. Their indentations and concave contours add a sense of depth and complexity to their appearance. Concave dodecagons are often utilized in artistic representations, structural designs, and decorative motifs.
6. Convex Dodecagons: Convex dodecagons have no interior angles greater than 180 degrees. They do not have any indentations and possess a regular outward appearance. Convex dodecagons are commonly found in architectural designs, decorative tiles, and structural elements where a sleek and aesthetically pleasing shape is desired.
Each type of dodecagon offers unique visual characteristics and functions. From the symmetry of regular dodecagons to the complexity of star-shaped or concave dodecagons, these polygon variations find applications in art, architecture, design, and decorative works. The versatility of dodecagons allows for creative explorations and the utilization of their distinct features in various fields and forms of expression.
Real-World Applications of Dodecagons
Dodecagons, with their distinctive twelve sides and angles, find practical applications in various real-world contexts. The unique properties and aesthetic appeal of dodecagons make them valuable in different fields and industries. Let’s explore some of the real-world applications of dodecagons.
1. Architecture and Design: Dodecagons can be seen in architectural designs, particularly in decorative elements and floor patterns. Their symmetric and balanced shape adds elegance and visual interest to building facades, windows, and interior spaces. Dodecagonal patterns, such as star-shaped dodecagons, are used in decorative motifs and artistic expressions.
2. Timekeeping Devices: Dodecagons are utilized in the design of timekeeping devices like clocks and watches. The twelve-hour division of time is often based on the dodecagonal shape, ensuring an even distribution of hours on the clock face. This application maintains accuracy and a consistent representation of time.
3. Sporting Equipment: In sports, dodecagons are incorporated into the design of balls and sporting equipment. For example, some soccer balls feature dodecagonal panels along with pentagonal panels. This arrangement ensures greater accuracy in ball movement, providing better control and stability during gameplay.
4. Flooring and Tiling: The regularity and symmetry of dodecagons make them suitable for tiling in flooring designs. Dodecagonal tiles can be arranged to create visually captivating patterns with seamless interlocking. These tiles are commonly used in entrances, foyers, and other areas where an eye-catching floor design is desired.
5. Industrial Applications: Dodecagons find applications in various industrial settings. They can be utilized in the design of machine parts, mechanical components, and architectural hardware. The geometric stability and symmetrical properties of dodecagons contribute to their effectiveness in providing structural support and facilitating precision in manufacturing processes.
6. Art and Design: Dodecagons offer artists and designers a unique shape to explore and incorporate into their creations. From sculptures to jewelry design, dodecagons can serve as a basis for intricate patterns and geometric forms. The aesthetic appeal of dodecagons enhances artistic expressions and adds a touch of sophistication to various designs.
7. Decorative Applications: Dodecagons are often used in decorative applications in interior design, furniture, and home decor. Their geometrically pleasing shape can be found in decorative accents, mirrors, lighting fixtures, and ornamental details. Dodecagons add a sense of elegance and refinement to spaces, creating a visually captivating ambiance.
From architecture and sports to industrial applications and artistic expressions, dodecagons have found a place in numerous real-world applications. Their aesthetic appeal, symmetrical properties, and mathematical significance make them valuable in various fields, contributing to functional and visually stunning designs.